Height and Distance

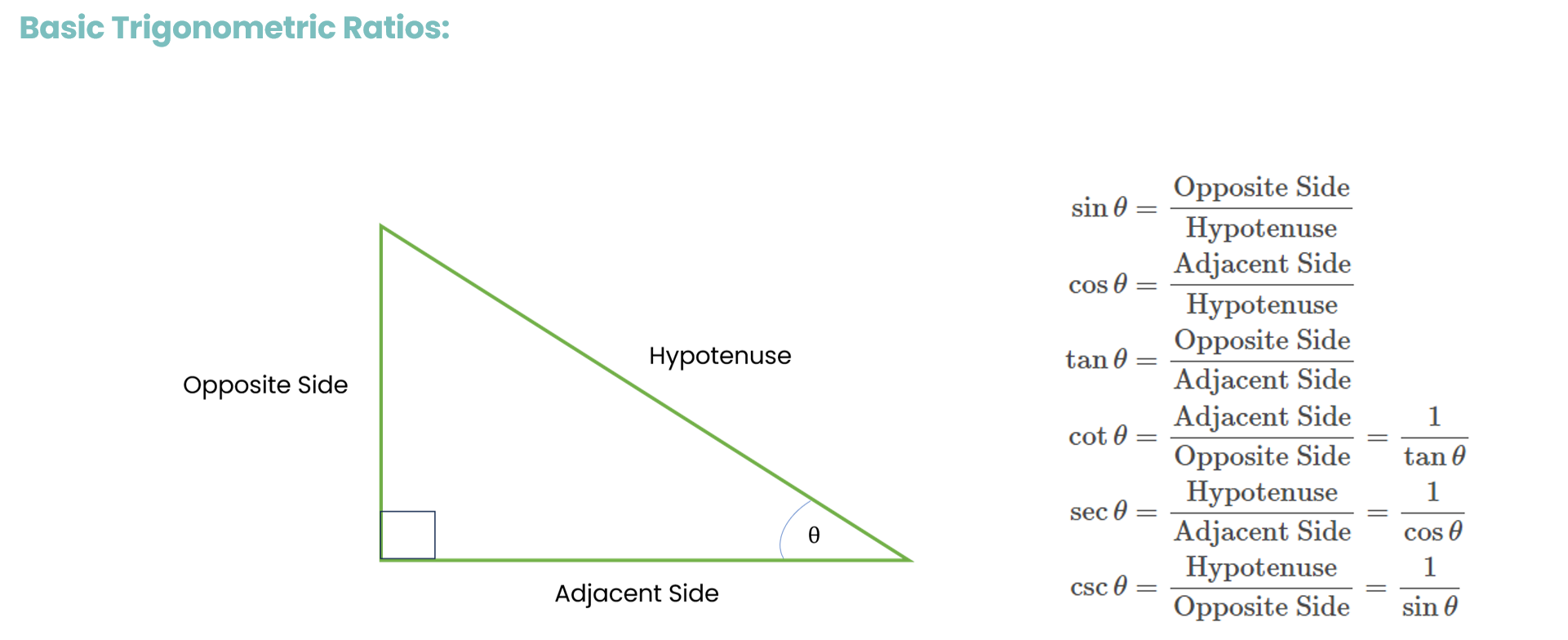
1. Basic Trigonometric Ratios

The basic trigonometric ratios are defined as follows:

$$\begin{array}{rl}\sin \theta & =\frac{\text{Opposite Side}}{\text{Hypotenuse}}\\ \cos \theta & =\frac{\text{Adjacent Side}}{\text{Hypotenuse}}\\ \tan \theta & =\frac{\text{Opposite Side}}{\text{Adjacent Side}}\\ \cot \theta & =\frac{\text{Adjacent Side}}{\text{Opposite Side}}=\frac{1}{\tan \theta }\\ \sec \theta & =\frac{\text{Hypotenuse}}{\text{Adjacent Side}}=\frac{1}{\cos \theta }\\ \csc \theta & =\frac{\text{Hypotenuse}}{\text{Opposite Side}}=\frac{1}{\sin \theta }\end{array}$$

---

2. Angle of Elevation

The angle of elevation is the angle between the horizontal line and the line of sight looking up to an object.

If a person is looking at the top of a tower from a distance, then:

$$\tan \theta =\frac{\text{Height of the Object}}{\text{Distance from the Object}}$$

3. Angle of Depression

The angle of depression is the angle between the horizontal line and the line of sight looking down to an object.

If a person is looking at the base of a tower from the top of another tower, then:

$$\tan \theta =\frac{\text{Height of the Object}}{\text{Distance from the Object}}$$

4. Height of the Object

To find the height of an object when the distance and the angle of elevation are known:

$$\text{Height}=\text{Distance}\times \tan \theta$$

5. Distance from the Object

To find the distance from the object when the height and the angle of elevation are known:

$$\text{Distance}=\frac{\text{Height}}{\tan \theta }$$

6. Relationship Between Angles and Sides in a Right-Angled Triangle

For a right-angled triangle with angle $\theta$, the sides are related by the Pythagorean theorem:

$${\text{Hypotenuse}}^2={\text{Opposite Side}}^2+{\text{Adjacent Side}}^2$$

Additional Formulas for Height and Distance

Finding Height Using Two Angles of Elevation

When the angles of elevation of the top of a tower from two points at a distance $d$ apart on a horizontal line and in the same vertical plane as the tower are $\alpha$ and $\beta$ ($\alpha >\beta$), then:

$$\text{Height of the Tower}=\frac{d\times \tan \alpha \times \tan \beta }{\tan \alpha -\tan \beta }$$

Finding Distance Using Two Angles of Elevation

If the height of a tower is $h$ and the angles of elevation from two points at a distance $d$ apart on a horizontal line and in the same vertical plane as the tower are $\alpha$ and $\beta$ ($\alpha >\beta$), then:

$$\text{Distance between the two points}=h(\frac{1}{\tan \beta }-\frac{1}{\tan \alpha })$$